take-the-deal analyzer
 go for the multi-millions or not?? see analysis of whether to take the deal or to try for the $1m. based on the trade-off of greed vs. need. go for the million.
go for the multi-millions or not?? see analysis of whether to take the deal or to try for the $1m. based on the trade-off of greed vs. need. go for the million.
based on the tv show, deal or no deal, how do you decide when player should take the deal? that decision should be based on the belief that the odds of coming out ahead are in your favor. just like in playing poker or video poker games. that probability and gambling factor is called the expected payoff, or ep.
our spreadsheet software calculates that figure and individual next level payoffs based on the actual case value chosen. this allows the player to see the probable new ep, and bank deal that is partially based on this figure. partially because the show must be entertaining and so the deal gets manipulated somewhat from the ep, and is generally a little lower. it answers: what happens if i picked the $200,000 case? or, the $10,000 case?
remember, it�s a game of chance where everything is possible, but not probable. there are no guarantees, just lots of fun.
you need to download the software in order to follow the following explanation on how to use take-the-deal analyzer. download the zip file. requires ms excel.
this is a free 30-day trial version. the standard version without any time constraint is available for just $10 us. for any questions, ask george.
this page viewed times.
note: you can have family and party fun at home by playing deal or no deal online at nbc. open take the deal analyzer as you play
|
|
|
|
|
|
|
|
|
|
when to take
the deal |
|
|
|
|
|
||
|
|
|
|
|
|
|
|
|
|
1. when the spread between the ep and deal
amounts are worthwhile and meaningful. |
|
||||||
|
this will also be indicated by the
bk fair game being a decent plus amount. |
|
|
|||||
|
|
|
|
|
|
|
|
|
|
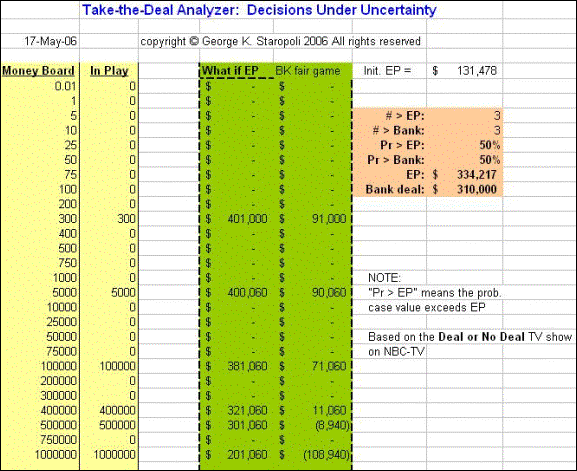
2. for example, loading the values from round
1 shown in the historical area, |
|
|
|||||
|
the deal is $310,000 with a 50% chance
of selecting amounts lower than the deal. |
|
||||||
|
selecting a lower amount would bring the
new deal somewhere in the $390,000 area, but usually |
|||||||
|
lower than the eps shown. is the gamble for a $80,000 increase worth
a possible decrease in the |
|||||||
|
new deal offer of about the same
amount? it could also be as low as $100,000
if the $1,000,000 |
|
||||||
|
amount is picked. |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
3. you must remember that the player is not
choosing the amount in his case, but trying to eliminate |
|||||||
|
those lesser amounts.
that is the objective of each choice whether to deal or to not deal. |
|
||||||
|
|
|
|
|
|
|
|
|
|
4. the two probablility, expectation to win
how much, questions are: |
|
|
|||||
|
a)
what's the chance of eventually eliminating all the cases less than
the highest |
|
||||||
|
amount shown, or $1,000,000 in
this instance? there are 6 choices
yet to be made in order to get |
|||||||
|
the $500,000. that comes to an 83% chance, after 6
choices, that you lose. |
|
||||||
|
if you win the 1 out of 6 chance
and pick the $1,000,000, you lose, not win! |
|
||||||
|
next round, there is a 1 of 5
chance to pick the $1,000,000 and lose, etc. |
|
|
|||||
|
b)
when should i accept the deal?
this is the important on-the-spot-decision discussed in (2) above. |
|||||||
|
there is a 50 -50 chance to beat
the bank, or to lower the next deal.
the player must look to the |
|||||||
|
next round deal amount that will
be offered. the player is not playing the lottery, but a game |
|||||||
|
of surviving each round until the very last, if there is a chance of winning much more than being |
|||||||
|
offered, or will be offered, by the bank. |
|
|
|
|
|||
|
for example, suppose the
player's highest win is only $50,000 at a 50% chance of beating the bank |
|||||||
|
deal of $35,000, with a new
down-side deal of $20,000. deal or
no-deal? that should be a deal! |
|||||||
|
take the money and run! |
|
|
|
|
|
||
|
in historical game 3, the bank
deal is greater than the ep. deal or no deal?
deal! |
|
||||||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|

